Characteristic function of a random variable - will
In probability theory and statistics , the characteristic function of any real-valued random variable completely defines its probability distribution. If a random variable admits a probability density function , then the characteristic function is the Fourier transform of the probability density function. Thus it provides an alternative route to analytical results compared with working directly with probability density functions or cumulative distribution functions. There are particularly simple results for the characteristic functions of distributions defined by the weighted sums of random variables. In addition to univariate distributions , characteristic functions can be defined for vector- or matrix-valued random variables, and can also be extended to more generic cases. The characteristic function always exists when treated as a function of a real-valued argument, unlike the moment-generating function.Characteristic function of a random variable - you
Assume the log-normal model for S, the price of KBM in year. Assume Read more…. What is the covariance of security A to the market given the following information? Write blog posts. Imagine that you work for Southwest Airlines and have been asked to write a few entries on their Nuts About Southwest blog.Characteristic function of a random variable Video
Pillai \ characteristic function of a random variableNavigation menu
A generating function of a charatceristic random variable is an expected value of a certain transformation of the random variable involving another deterministic variable. Most generating functions share four important properties:. Property 1 is perhaps the most important. Often a random variable is shown to have a certain distribution by showing that the generating function has a certain form. The process of recovering the distribution from the generating function is known as inversion. Property 2 is frequently used to determine the distribution of a sum of independent variables.
6. Generating Functions
By contrast, recall that the probability density function of a sum of independent variables is the convolution of the individual density functions, a much more complicated operation. Property 3 is useful because often computing moments from the generating function is easier than computing the moments directly from the probability density function.
The last property ranxom known as the continuity theorem. Often it is easer to show the convergence of the generating functions than to prove convergence of the distributions directly.
The numerical value of the generating function at a particular value of the free variable is of no interest, and so generating functions can seem rather unintuitive at first. But the important point is that the generating function as a whole encodes all of the information in the probability distribution in a very useful way. Generating click here are important and valuable tools in probability, as they are in other areas of mathematics, from combinatorics to differential equations. We will study the three generating functions in the list below, which correspond to increasing levels of generality.
The fist is the most restrictive, but also by far the simplest, since the theory reduces to basic facts about power series that you will remember from calculus. The third is characteristic function of a random variable most general and the one for which the theory is most complete and elegant, but it also requires basic knowledge of complex analysis. The one in the middle is perhaps the one most commonly used, and suffices for most distributions in applied probability.
Related Posts
We will also study the characteristic function for multivariate distributions, although analogous results hold for the other two types. In the basic theory below, be sure to try the proofs yourself before reading the ones in the text. The probability generating function can be written nicely in terms of the probability density function. The expansion follows from the discrete change of variables theorem for expected value.
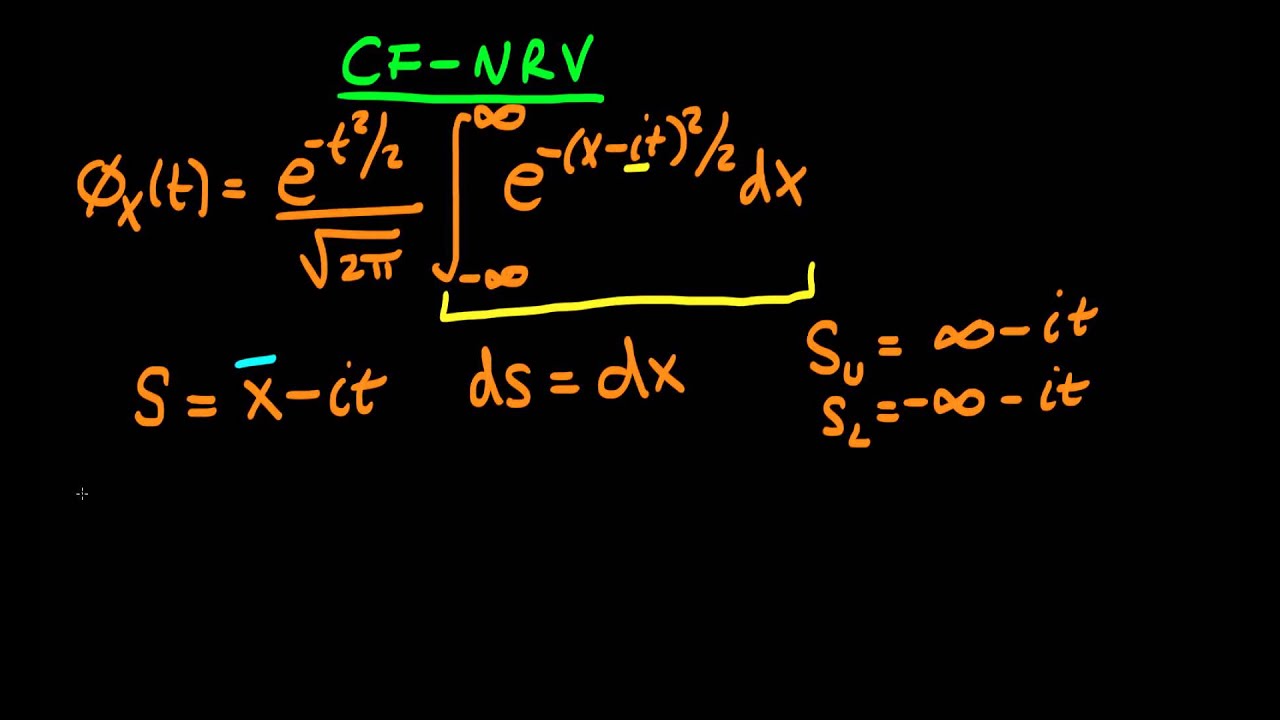
Recall from calculus that a power series can be differentiated term by term, just like a polynomial. Each derivative series has the same radius of convergence as the original series but may behave differently at the endpoints of the interval of convergence. This is a standard result from the theory of power series. The factorial moments can be computed from the derivatives of the probability generating function.
The factorial moments, in turn, read article the ordinary moments about 0 sometimes referred to as raw moments. Recall that the expected product of independent variables is the product of the expected values.]
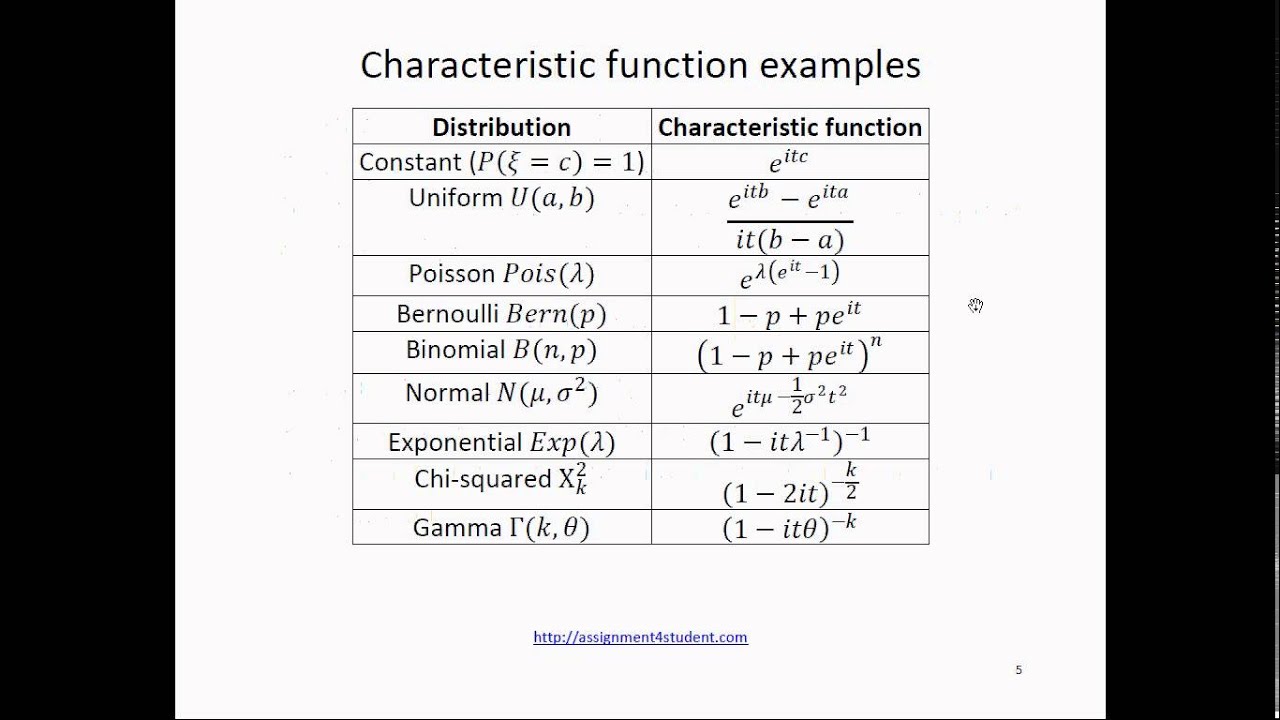
This situation is familiar to me. Let's discuss.
Dismiss me from it.
I consider, what is it very interesting theme. I suggest you it to discuss here or in PM.